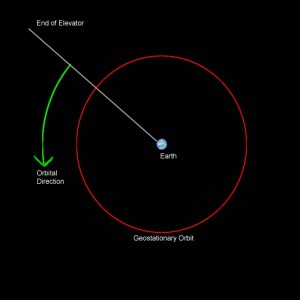
 You’ve heard of space elevators, right? You know, it’s that cable that hangs down to Earth from geostationary orbit and stretches out another 35,786 kilometers past that to balance it all out. Down here on Earth, we’re experiencing one Earth gravity, 9.8 m/s2, aka “1 g”. And up at the center, in geostationary orbit, we’re weightless. And at the far end, we’re experiencing full Earth gravity again, only pointed outwards due to centripetal force, right?
You’ve heard of space elevators, right? You know, it’s that cable that hangs down to Earth from geostationary orbit and stretches out another 35,786 kilometers past that to balance it all out. Down here on Earth, we’re experiencing one Earth gravity, 9.8 m/s2, aka “1 g”. And up at the center, in geostationary orbit, we’re weightless. And at the far end, we’re experiencing full Earth gravity again, only pointed outwards due to centripetal force, right?
Wrong.
I’ve seen this crop up in a few SF stories involving space elevators, and it seems so natural that I assumed they were correct. It makes sense, after all. The bottom is being pulled down at one Earth gravity. To balance out, the other end also has to be getting pulled up at one Earth gravity. It makes sense, but it’s wrong.
Why? The key is that Earth’s gravity is stronger at the surface than in the increasingly higher orbital altitudes. Somewhere in the back of your head, you’re starting to remember something called the Inverse Square Law, aren’t you? That’s right. Like many physical phenomena, their effects decreases with the square of the distance. If you’re twice as far away, you only feel one fourth the effect.
What does that mean for our space elevator? It means that at its center, we are not balancing 1 g of gravity with 1 g of centripetal force. Rather, we’re balancing the decreased gravity from the distant Earth with an equivalent amount of centripetal force. How much gravity is there at that distance? Let’s do the math.
The formula for gravity at a distance is:
g = GM / r2
… where G is the gravitational constant, M is the mass of the body (i.e. Earth), and r is the radius of the orbit. The value of the product GM is known to high precision at 398,600.4418 km3/s2, and the geostationary orbit radius is 42,164km — 6300 of which are between the Earth’s center and surface. So at that altitude, the gravitational attraction of the earth is 0.224 m/s2, or a mere 2.28% of the gravity we experience on the surface of the Earth at the base of the space elevator.
But what does that say about the centripetal force we experience out at the far end? Well, centripetal acceleration is defined by the formula
a = w2 * r
…where w is the angular velocity in radians per second, and r is the radius of the circle we’re turning in. Since we’re still staying over the same point on earth, the angular velocity is (2 * pi) per day or roughly 0.0000729 radians per second, and our radius is about 77,950km, i.e. twice the geostationary altitude plus the radius of the Earth itself. And being swung around at that far-flung distance will net us a grand total centripetal acceleration of… 0.41 m/s2.
But we’re not quite done, because even though we’re being flung around, we’re still feeling some gravity pulling us back to the Earth. At that distance, it’s only 0.065 m/s2, but that does drop us down to 0.35 m/s2, or a mere 3.6% the surface gravity of the Earth.
You thought the Apollo astronauts looked bouncy on the Moon? They were over four and a half times heavier than they would be at the end of the elevator’s cable. It’s enough to keep things on the floor, but it’s not enough to feel very strongly.
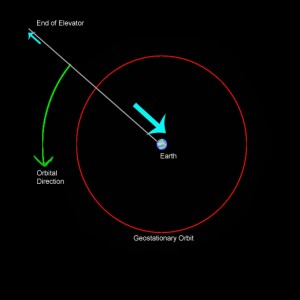 This also points out an interesting problem with balancing the elevator. After all, it’s that balance between the Earth pulling it down at the spin flinging it out that keeps it in place. But as we’ve seen, the bottom of the cable is being pulled down towards the Earth at 9.8 m/s2 while the other end is only being pulled out at a mere 0.35 m/s2. Thus, while the top and bottom halves of the cable may have the exact same mass, the bottom half is heavier. That is, it is being pulled upon with more force.
This also points out an interesting problem with balancing the elevator. After all, it’s that balance between the Earth pulling it down at the spin flinging it out that keeps it in place. But as we’ve seen, the bottom of the cable is being pulled down towards the Earth at 9.8 m/s2 while the other end is only being pulled out at a mere 0.35 m/s2. Thus, while the top and bottom halves of the cable may have the exact same mass, the bottom half is heavier. That is, it is being pulled upon with more force.
The solution to this was given when these space elevators were first proposed, and that was to put a ballast weight at the far end of the cable. If it’s massive enough, it does not even need to be at double the geostationary altitude. It could be closer to the Earth, but since it would still be above geostationary orbit, it would be pulling with all of its weight (its mass times its centripetal acceleration) outwards. Thus, you can still balance out the space elevator and keep it floating serenely in the sky.
Ahh, I hear you saying, but what if we just made the cable longer? After all, the further out the cable goes, the centripetal acceleration acting on it goes up. Maybe if we made it long enough so that the far end did experience one full g of centripetal acceleration, there would also be enough force from the mass of that longer cable to balance it out. Maybe there’s still hope for some kind of mirrored gravity at either end of the cable?
Unfortunately, no, it doesn’t work.
The root of the problem is that while gravity drops off with the square of the distance from the Earth, the centripetal acceleration builds linearly with the distance. To experience one gravity at the far end of the cable, being swung around us once per day, the cable would have to be 1.84 million kilometers long.
There are two problems with such a long cable. The first is that the orbit of the moon is only about 384,000 kilometers. While a space elevator on Mars would face a similar problem with the small moons there, it has been suggested that the moons are small enough that well-timed wave maneuvers (like plucking a taut wire) would allow the elevator there to dodge the moons. However, Earth’s moon is 156 times wider than the largest of Mars’ moons, and even if its slow-moving body could be dodged, it would be impossible to dodge its gravity which would take the math into even crazier areas.
But the bigger problem goes back to that disparity between the two forces’ relationship to radius. While the gravitational acceleration acting on the bottom of the cable drops away rapidly as it rises from Earth’s surface, the acceleration acting on the far end of the cable as we back away from the end would only decrease linearly. So, while the mass of the “top half” of the cable would be only fifty times as massive (at a minimum), the force acting on it (the mass of each part times the centripetal acceleration acting on it) would be over a thousand times as great as the force of the lower half being pulled down to the Earth.
Even if it was made from a material cable of withstanding that much force, there is no way we could secure it to the surface of the Earth. It would be plucked from its docking station like an unwanted nose hair and flung into space like… well, like an unwanted nose hair.
So keep the solar system free of nasty million-kilometer cables, and don’t insist on 1 g of centripetal acceleration at the end of your space elevators.
 When the planet is much closer to one star than the other, the distant star does not have much gravitational influence on the planet’s orbit. It can orbit the smaller star in a nice circular orbit, almost as though the other star weren’t there at all. Well, it looks like a circular orbit from the point of view of the nearby star. From the point of view of the system’s center of gravity, it’s more akin to a spirograph. (And yes, I know that this dates me, but spirographs were cool!)
When the planet is much closer to one star than the other, the distant star does not have much gravitational influence on the planet’s orbit. It can orbit the smaller star in a nice circular orbit, almost as though the other star weren’t there at all. Well, it looks like a circular orbit from the point of view of the nearby star. From the point of view of the system’s center of gravity, it’s more akin to a spirograph. (And yes, I know that this dates me, but spirographs were cool!) Here, instead of Tatooine’s double-sunset, you’ll have half the year with no night at all. Sure, one sun will set, just as the other one is coming up. I imagine that will be summer, regardless of the planet’s tilt, because overnight lows only come when there is night. It might make for an interesting place to live, but the seasons could be a little intense.
Here, instead of Tatooine’s double-sunset, you’ll have half the year with no night at all. Sure, one sun will set, just as the other one is coming up. I imagine that will be summer, regardless of the planet’s tilt, because overnight lows only come when there is night. It might make for an interesting place to live, but the seasons could be a little intense. Here, your orbit is stable, close to circular, and any seasons you have will be due to axial tilt, not varying proximity to the great fiery balls in the sky. Plus, you know… the infamous double-sunset.
Here, your orbit is stable, close to circular, and any seasons you have will be due to axial tilt, not varying proximity to the great fiery balls in the sky. Plus, you know… the infamous double-sunset.


